Fifecge
| Ēacnung: Forþan þe þām nīwegemyndum þāra rīmcræften ƿēron, sume þisse rēdes synd on Nīƿenglisce. |
| Fifecge | |
|---|---|
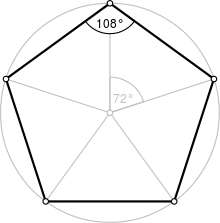 Ǣn samaflanc fifecge | |
| Rindenes | 5 |
| Sclæfligruna | {5} |
| Oferside | Manigfult |
| Twēoflaedig horn | 108° |
Sēo fifecge (Niwenglisc: pentagon) oþþe fifhyrne ġesceap is sēo ġesċeap ƿiþ fif ecgeġen.
Samaflanc fifecges
adihtanSēo samaflanc fifecge hæfde sēo sclæfligruna æf {5} ond innyrdigfealds æf 108°.
Sēo samaflanc fifecge hæfde fif rindenes æf ābēodendlīcum gelīċnes, and ymbhrǣdlic gelīċnes æf ordre 5 (þurh 72°, 144°, 216° and 288°). Þē gegendlihts æf sēo ūtbendan gewunlic fifecge are in þē golden hlutord to hits rindenes. Giefan hits side lengð hits stēapne (feornes fram ān side to þē wiðerlang hwyrftstān), wīdnes (Niwenglisc: width) (feornes betwēonum tƿā feorrum āgesliten ords, hwic īsgelīcen þē gegendliht lengð ) and ymbhrēodanfex are giefan by:
Sēo oferside æf sēo ūtbendan gewunlic fifecge ƿiþ side lengð is giefan by
If þē ymbhrēodanfex æf sēo gewunlic fifecge is giefan, hits eċġ lengð is befunden by þē ǣwednes
and hits oferside is
siþþan þē oferside æf þē ymbwrāted hring is þē gewunlic fifecges fills nēah 0.7568 æf hits ymbwrāted hring.
Derivation æf þē oferside gerihtlǣcung
adihtanÞē oferside æf ænig gewunlic ġesceap is:
ƿǣr P is þē ymbhāt (Niwenglisc: perimeter) æf þē ġesceap, and r is þē onhrēodanfex (gemænelīceg þē apothem). Underbēogenden þē gewunlic fifecges wurðscipes fore P and r giefas þē gerihtlǣcung
ƿiþ sīde lengð t.
Onhrēodanfex
adihtanAnlīċ to ǣġhwelċ gewunlic ūtbendan ġesceap, þē gewunlic ūtbendan fifecge hæfde an inwrāted hring. Þē apothem, hwic is þē hrēodanfex r æf þē inwrāted hring, æf sēo gewunlic fifecge is geferian to þē sīde lengð t by
Strēowas fram þē ymbwrāted hring to þē ƿēohpunctu
adihtanLīciġe ǣġhwelċ gewunlic ūtbendanġesceap, þē gewunlic ūtbendan fifhyrne hæfde sēo ymbwrāted hring. Fore sēo gewunlic fifhyrne ƿiþ æfterfylgendlic ƿēohpunctu A, B, C, D, E, if P is ænig ord on þē ymbhring betwēonum ord B and C, þēn PA + PD = PB + PC + PE.
Ord in plane
adihtanFore æn wyllfull ord in þē plane æf sēo gewunlic fifhyrne ƿiþ ymbhrēodanfex , hwæs feornesse to þē gemǣnellǣhtwyrft æf þē gewunlic fifhyrne and hits fif ƿēohpunctu are and gehwādorlīce, ƿē habbað[1]
If are þē feornesse fram þē ƿēohpunctu æf sēo gewunlic fifhyrne to ænig ord on hits ymbhringġesceap , þēn [1]
Geometrical bytles
adihtanÞē gewunlic fifhyrne is edlēhendlic ƿiþ færanfæþm and straighteċġ, as 5 is sēo Fermat prime. Sēo gerifl æf ǣrendweges are cnāwen fore bytleden sēo gewunlic fifhyrne. Sum are gedǣled neoþera.
Ricemunden ǣrendweg
adihtanĀn ǣrendweg to bytle sēo gewunlic fifhyrnein sēo giefan hringġesceap is æfwrāted by Ricemund[2] and ġēond gedǣled in Cromwell's Polyhedra.[3]
Þē top scēadwelle geswutelaþ þē edlēhendlic brūcaned in Ricemunden ǣrendweg to cræfte þē sīde of þē inwrāted fifhyrne. Þē hringġesceap geƿissung þē fifhyrne hæfde ord hrēodanfex. Hits middel is befērēd æt ord C and sēo middumord M is gemearcod hālfweg be hits hrēodanfex. Þis ord is tōgæderebunden to þē ytemest swāupp upper þē middel æt ord D. Hyrne CMD is twafealden, and þē twafoldere samētanes þē uppīweard stefn æt ord Q. Sēo wīdnesline þurh Q samētanes þē hringġesceap æt ord P, and strēowa PD is þē þearf sīde æf þē inwrāted fifhyrne.
Tō bestimma þē lengð æf þis side, þē tƿā rihtþrīhyrnes DCM and QCM are geƿeorþod neoþera þē hringġesceap. Brūcende Pīthagoras þeoruma and twa rindenes, þē underwige æf þē lengra þrīhyrnes is befunden as . Sīde h æf þē læssa þrīhyrne þēn is befunden brūcende þē twāfealdhyrne gerihtlǣcung:
ƿǣr cōsīne and sīne æf ϕ are cnāwen fram þē lengra þrīhyrne. Þē geswutelung is:
If DP is sōðlīce þē sīde æf sēo gewunlic fifhyrne, , so DP = 2 cos(54°), QD = DP cos(54°) = 2cos2(54°), and CQ = 1 − 2cos2(54°), hwic īsgelīcen −cos(108°) by þē cōsīne twāfeald gerihtlǣcung. Þis is þē cōsīne æf 72°, hwic īsgelīcen as gewilnode.
Ceorlēl hringġesceapu
adihtan- Heafodgewrit: Ceorlēl hring
Sēo Ceorlēl hringġesceap wæs crǣften as sēo gemetgemostric ǣrendweg to ġefrīgean þē wyrtwela æf sēo fēoworhyrnum efenunga.[4] Þis ǣrendgewritlīce to sēo procedure fore bytleden sēo gewunlic fifhyrne. Þe stōpen are ǣs fallowen:[5]
- Drage sēo hringġesceap in hwic to inwrāt þē fifhyrne and mark þē middelord O.
- Drage sēo wīdnesline þurh þē middel æf þē hringġesceap. Mark þē left samētanum ƿiþ þē hringġesceap as ord B.
- Bytle sēo uppīweard line þurh þē middel. Mark ān samētanum ƿiþ þē hringġesceap as ord A.
- Bytle þē ord M as þē middumord æf O and B.
- Drage sēo hringġesceap middeled æt M þurh þē ord A. Mark hits samētanum ƿiþ þē wīdnesline (inside þē frumlic hringġesceap) as þē ord W and hits samētanum outside þē hringġesceap as þē ord V.
- Drage sēo hringġesceap æf hrēodanfex OA and middel W. Hit samētanumes þē frumlic hringġesceap æt tƿā æf þē ƿēohpunctu æf þē fifhyrne.
- Drage sēo hringġesceap æf hrēodanfex OA and middel V. Hit samētanumes þē frumlic hringġesceap æt tƿā æf þē ƿēohpunctu æf þē fifhyrne.
- Þē fifþ hwyrftstān is þē rihtmǣst samētanum æf þē wīdnesline ƿiþ þē frumlic hringġesceap.
Steps 6–8 are gemænelīce to þē fylgende feorðunga, getǣled in þē hrinanġemāting:
- 6a. Bytle ord F as þē middumord æf O and W.
- 7a. Bytle sēo uppīweard line þurh F. Hit samētanes þē frumlic hringġesceap æt tƿā æf þē ƿēohpunctu æf þē fifhyrne. Þe þird hwyrftstān is þē rihtmǣst samētanum æf þē wīdnesline ƿiþ þē frumlic hringġesceap.
- 8a. Bytle þē ōþer twa ƿēohpunctu brūcende þē færanfæþm and þē lengð æf þē hwyrftstān befunden in step 7a.
Ewcleoden ǣrendweg
adihtanSēo gewunlic fifhyrne is towearplic brūcende sēo færanfæþm ond streċċaneċġ, ǣġhwæþer by inscribing ān in sēo giefan hringġesceap or bytleden ān on sēo giefan eċġ. Þis gecynd wæron ǣfwrāted by Ewcleod on his bōc, Ādalen circa 300 BC.[6][7]
Lychamlic ƿeorc ǣrendweges
adihtan- Sēo gewunlic fifhyrne magan biþ cræftum fram iust sēo stripe æf papyr by tying an offerhond cnotta ōntū þē stripe and carefully flattæneg þē cnotta by pulliġen þē ends æf þē papyr stripe. Fealdeg ān æf þē endes bæc offer þē fifhyrne willa sċēawaþ sēo fīfhyrnedesteorra when bæclīhtte.[8]
- Bytle sēo gewunlic seoxhyrne on stīf papyr or bord. Twāfeald ǣlang þē þrī diameters betwēonum wiðerlang ƿēohpunctu. Cutt fram ān hwyrftstān tō þē middel tō macen an samaflanc þrīhyrneum flēapan. Gelfan þis flēapan underneoþan hits nēahgebyr to cræften sēo fifhyrnum þrīhyrnetorr. Þē baas æf þē þrīhyrnetorr is sēo gewunlic fifhyrne.
Ȝelīċnes
adihtanÞē gewunlic fifhyrne has Twyflax5 gelīċnes, ordre 10. Siþþan 5 is sēo formarim þǣr is ān underflocc ƿiþ twyflax gelīċnes: Dih1, and 2 cyclic flocc gelīċnese: Z5, and Z1.
Þas 4 gelīċnese cunnan biþ geseon on 4 geondweard gelīċnese on þē fifhyrne. Iohannes Cōnweg ingraves þas by sēo rūna and flocc ordren.[9] Full gelīċnes æf þē gewunlic form is r10 and no gelīċnes is ingraved a1. Đā twyflaxen gemetgemostras syndon dælode be þǣm hwæðer hī faraþ þurh wēohsēoꝛpunga (d fore gegendliht) or eċġen (p fore perpendiculars), and i hwænne ābēodendlīc rindenes bēah þurh bēgen eċġen and wēohpunctu. Hringlic gelīċnese in þē middel stēap are ingraved as g fore hiera midlig gyration ordren.
Gæt wēnġungflocc geliċnes hlēomāþe wēlġað wān oþþe mā dæġēð ān wīnōð ofer unġewōðelicum ƿyrmbēam. Ānlȳċ þæt g5 ƥēodhlēomāþ hæfð nān frīġedēoƿu āc mæġ bēon gesēon swā bewundenum hrēod.
Gewunlic fīfhyrnedesteorra
adihtan- Heafodgewrit: Fīfhyrnedesteorra
Sēo fīfhyrnedesteorra is sēo gewunlic steorra fifhyrne. Hits Sclæfliruna is {5/2}. Hits rindenes form þē gegendlihts æf sēo gewunlic ūtbendan fifhyrne – in þis gecynd þē rindenes of þē tƿā fifhyrnen are in þē golden hlutord.
Fruman
adihtan- ↑ 1.0 1.1 Meskhishvili, Mamuka (2020). "Cyclic Averages æf Regular Polygons and Platonic Solids". Communications in Mathematics and Applications 11: 335–355. https://www.rgnpublications.com/journals/index.php/cma/article/view/1420/1065.
- ↑ Richmond, Herbert W. (1893). "A Edlēhendlicen fore sēo Regular Polygon of Seventeen Sides". The Quarterly Journal of Pure and Applied Mathematics 26: 206–207. https://books.google.com/books?id=bcMKAAAAIAAJ&pg=PA206.
- ↑ Peter R. Cromwell (22 July 1999). Polyhedra. ISBN 0-521-66405-5.
- ↑ Eric W. Weisstein (2003). CRC concise encyclopedia of mathematics, 2nd, CRC Press. ISBN 1-58488-347-2.
- ↑ DeTemple, Duane W. (Feb 1991). "Carlyle circles and Lemoine simplicity æf polygon constructions". The American Mathematical Monthly 98 (2): 97–108. doi:10.2307/2323939. Archived from the original. Bysen:Citation error. https://web.archive.org/web/20151221113614/http://apollonius.math.nthu.edu.tw/d1/ne01/jyt/linkjstor/regular/1.pdf#3.
- ↑ George Edward Martin (1998). Geometric constructions. Springer. ISBN 0-387-98276-0.
- ↑ (2008) Euklid's Elements of Geometry, Book 4, Proposition 11. ISBN 978-0-615-17984-1.
- ↑ Mathematical Models by H. Mærtyn Cundīg and A.P. Rollett, second edition, 1961 (Oxnaford University Press), p. 57.
- ↑ John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, (2008) The Symmetries æf Things, ISBN: 978-1-56881-220-5 (Chapter 20, Generalized Schaefli symbols, Types of gelīċnes æf sēo polygon pp. 275-278)
| Gesceapu | |
|---|---|
| Þrīhyrn | Cēplere • Ġetælhyrne • Samaflanc • Sċeorthyrne • Riht • Twāflanc • Ƿilnunȝ |
| Fēowerecge | Antiparallelogram • Bicentric • Crossed • Cyclic • Equidiagonal • Ex-tangential • Harmonic • Isosceles trapezoid • Kite • Orthodiagonal • Parallelogram • Langfēowerecge • Right kite • Right trapezoid • Rhombus • Fēowertynefeald • Tangential • Tangential trapezoid • Ungewunelic |
| By flanccounten (1–10) |
Ānecge (1) • Twāecge (2) • Þrīecge (3) • Fēowerecge (4) • Fifecge (5) • Seoxecge (6) • Seofonecge (7) • Eahtaecge (8) • Nigonecge (9) • Tīenecge (10) |
| By flanccounten (11–20) |
Endleofanecge (11) • Tƿelfecge (12) • Þrēotīeneecge (13) • Fēowertīeneecge (14) • Fīftīeneecge (15) • Seoxtīeneecge (16) • Seofontīeneecge (17) • Eahtatīeneecge (18) • Nigontīeneecge (19) • Tƿēntiġecge (20) |
| Steorraġesceapu | Fīfhyrnedesteorra • Pentagram • Hexagram • Heptagram • Octagram • Enneagram • Decagram • Hendecagram • Dodecagram |